The Schelling segregation model

Many cities around the world have racially segregated neighborhoods: minorities tend to be concentrated in particular neighborhoods instead of being spread out. The degree to which this occurs differs a lot from place to place, but the fact that segregation exists is undisputed and a frequent topic of debate. Even if you're not familiar with all of the data, you probably heard of Chinatown and Little Italy.
Racially segregated neighborhoods are a frequent topic of discussion in relation to the scary forces of polarization, inequality and racism. The reasoning typically goes as follows: if you are always surrounded by like minded people, chances are that over time you will share the opinion and income level of those around you. In a liberal and capitalist world, free information flow and diversity is important. Moreover, highly segregated neighborhoods might both be caused by, as well as stimulate, racism.
In order to understand to what extent racial segregation should be minimized, it is important to see where this segregation comes from.
An agent-based segregation simulation
Thomas Schelling, legendary storytelling economist, thought of a simple model which could explain how segregation evolved. Ultimately, the model:
"demonstrates that, in the multicultural context, seemingly mild preferences for living with similar neighbors carry the potential to be strong determinants for own race selectivity and residential segregation."
So, how does the model work?
"The original Schelling agent model was disarmingly simple in its construction (2, 3). It posited that an agent, a model representation of a household that could be white or black, preferred to be on a square on a checkerboard in which half or more of the eight adjacent neighbors were of a similar color. In the economic context, this was seen as having utility one compared with having utility zero. Schelling used simple simulations based on such hypothetical preference schedules to show that the adjustments of individual households responding to changes in composition on the checkerboard invariably lead to complete segregation (3)."
In each iteration of the above simulation, a household decides whether or not to move to another square in order to live in a more preferable neighborhood. When repeated over time, clusters of similar households emerge.
Now, for a model to hold predictive power, not just the output (level of segregation) needs to be correct, but it needs to coincide with certain input (people's preferences). And indeed, when asked, households from any racial background do typically favor residing with households of similar color!
"The Schelling model was critical in providing a theoretical basis for viewing residential preferences as relevant to understanding the ethnic patterns observed in metropolitan areas. Furthermore, its implications have been buttressed by accumulated findings on preferences in multicultural settings which show that all major racial and ethnic groups hold preferences that are as strong as or stronger than the relatively mild preferences Schelling considered in his original two-group formulation."
Now the three bad characteristics mentioned above (polarization, inequality and racism) may certainly be present in many facets of modern society. But it is not at all clear that these are causing racially segregated neighborhoods:
"‘‘Without any discriminatory behavior in the housing market a slight preference for like-color neighbors . . . can give rise to a high level of residential segregation and cause it to persist’’"
"Zhang established this for the two-color case with a preference for own color by one group and neutral preferences by the other group by drawing on the theory of stochastic dynamical systems and the method of agent modeling. Building on work in evolutionary game theory, he showed that even slight asymmetry in residential preferences between two groups will produce endogenous segregation. The basic model considers a set of n neighbors, either four in the rook’s case, or eight in the queen’s case, on a checkerboard lattice. Each of the locations may have a black agent or a white agent, or may be vacant.
"Under the conditions outlined earlier, Zhang shows that S, the set of all states that maximizes the sum of all agents’ utilities, is stochastically stable."
However, these preferences are not equal among different groups of people:
"An early study of Detroit used survey flash cards depicting different racial residential neighborhood combinations to establish that blacks generally preferred neighborhoods that were half black and half white, but whites generally preferred neighborhoods that were nearly all white (16). Subsequent studies of other metropolitan areas replicated this finding (17, 18) and also documented similar patterns in the preferences of other racial and ethnic groups (9)."

"There is a less obvious basis for linking preferences to segregation that has been termed ‘‘the paradox of weak minority preferences’’ (15). Setting whites’ preferences aside completely, minority preferences for 50/50 neighborhoods are rarely compatible with integration. Citywide integration obtains when all neighborhoods match the ethnic mix of the city. But few metropolitan areas have ethnic mixes that correspond to minority preferences for neighborhood ethnic mix. So the thought experiment of arranging neighborhoods to match blacks’ residential preferences for 50/50 racial mix does not lead to low segregation scores. To the contrary, for most metropolitan areas, the exercise produces substantial segregation (15)."
Beyond race: segregation is all around us
Although segregation between black and white populations is a canonical example, segregation can and does occur for any characteristic. People may have slight preferences to live and work with those of the same educational background, socio-economic situation, gender, skillset, etc. etc. And all of those nuances are taken into account in more recent simulations:
"advances in computing capabilities make it more feasible than ever to use simulation approaches to explore how residential outcomes vary over a wide range of increasingly complex and realistic model inputs (13, 14). For example, a recent model incorporates the role of multiple types of preferences, multiple ethnic groups, urban and demographic conditions, and intergroup inequality in socioeconomic status (15). Models of this sort expand our ability to investigate the residential patterns that emerge when agent choices are guided by different preference distributions, including both hypothetical distributions and ones fashioned after results from survey studies."
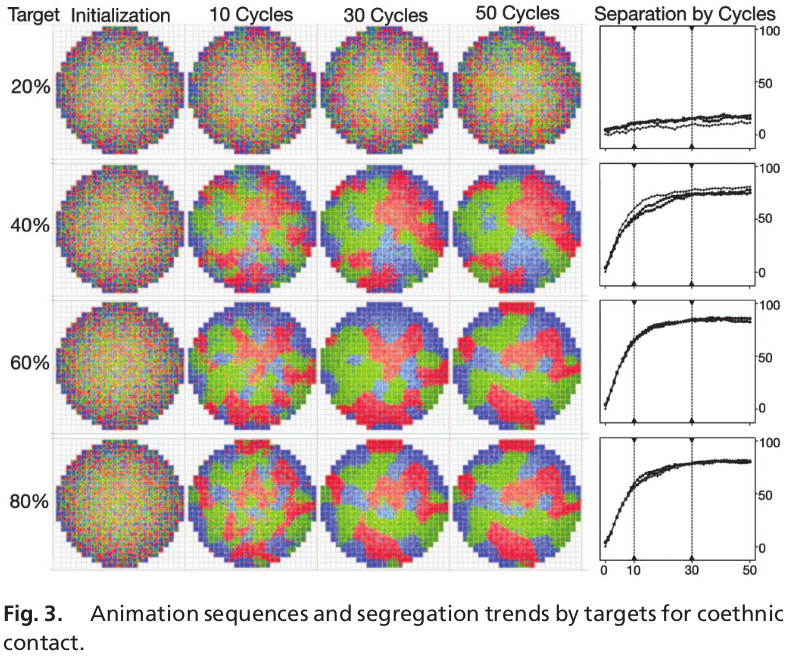
In this week's paper, the SimSeg model is used to run simulations. The original code, written in Delphi, isn't available anymore but various alternative tools exist. Presumably modern general-purpose agent based modeling tools as discussed in the previous editions of Equilibria Club must be able to help out. The SimSeg model work as follows.
"In brief, it creates housing units of varying quality and places them at residential locations across the city. It then populates this virtual city with households of different ethnic and socioeconomic status (income) and distributes them randomly to housing units. Ethnic segregation in the city thus initially reflects only random departure from even distribution. During the simulations households move according to the rules of the model by making residential choices guided by three separate and independent preference concerns: goals for housing quality, goals for neighborhood income, and goals for neighborhood ethnic composition. The program calculates segregation scores over the course of the simulation so trends in segregation can be assessed quantitatively and qualitatively based on graphical representations of the city’s residential distributions."
The following two mathematical formulas provide the basis for measuring segregation:
(1) The variance ratio:
\[ V = 1 - \cfrac{f_m}{N} \]Where f_m is the frequency (number of cases) of the mode and N is the total number of cases. In a population of M types, this is computed for each of the M possible two-group comparisons.
(2) The dissimilarity index:
\[D = \sum_{i=1}^N {| \cfrac{black_i}{black_{total}} - \cfrac{white_i}{white_{total}} |} \]"Group differences in socioeconomic standing have little impact on the segregation dimension of uneven distribution (which V measures), but minority socioeconomic disadvantage does serve to concentrate minority populations in high-poverty areas in the central city (15). In terms of potential social implications, it is obvious that this would foster disproportionate minority expo- sure to crime and other social problems found in high-poverty areas."
"The most powerful finding from this is that there is little evidence of extensive integration among any combination of ethnic groups resulting from residential choices guided by ethnic preferences. As in real cities, minorities are segregated not only from the majority group, but also from each other. This pattern is not predicted by theories of housing discrimination by whites."
Policy implications
In today's interconnected world, where people are sharing their personal information more widely than ever before, the Schelling Segregation model implies that we'll see more segregation as we get more information about our own and other people's characteristics.
Although polarization and socio-economic inequality between racial groups is a constant topic of debate, the typical range of policy recommendations I have seen focus on trying to ban or limit racial discrimination. And although discrimination is certainly a fact of life in many institutions, it is important to tackle systemic and deep causes to create a more equal world.
"Practices of legal, institutional race exclusion have been substantially dismantled, but stereotypes and prejudice remain and can promote residential separation in the absence of housing discrimination."
So, can we use policy or tools to change how people's preferences are formed?
Perhaps a progressive dictator can nationalize a version of Tinder and make its use mandatory. Or perhaps, we have to accept and learn to deal with segregation as a fact of life, and decrease our polarization, inequality and racism despite the existing segregation because fighting segregation in a free world is an asymmetric battle: a little bit of preference towards same can go a long way in forming segregation.
